

Fourier Analysis of a Square-Wave Grating


If you are not clear about what a square-wave grating is, you might want to read the tutorial that gives a basic description of gratings before continuing.
Fourier Analysis is a mathematical procedure used to determine the collection of sinewaves (differing in frequency and amplitude) that is neccessary to make up the square-wave pattern under consideration. Take, for example, one cycle of a square-wave which is graphed in Figure 1. This graph shows how luminance or light level changes over position as it falls across the surface of an object. Clicking on Figure 1 will bring down a square-wave made up of several cycles so that you can see what it would look like.
Below, Figure 2 shows a sinewave with the same size cycle as the square-wave shown above. Clicking on this figure will bring down a sinewave grating so that you can see what several cycles would look like.
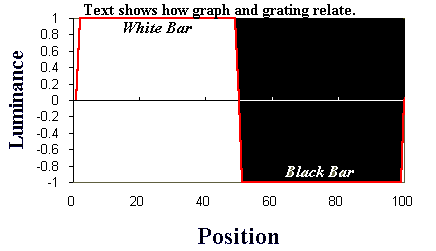 Figure 1.
A graph of a square-wave grating showing luminance as a function of position. Figure 1.
A graph of a square-wave grating showing luminance as a function of position. |
 Figure 2. A graph of a
sinewave grating showing luminance as a function of position. Figure 2. A graph of a
sinewave grating showing luminance as a function of position. |
Notice that even adding this one sinewave (called the fundamental because it is the lowest frequency and has the biggest amplitude) already gives the basic shape of the square-wave grating. The size of the bars and the contrast of the bars are already basically visible. What is lacking are the sharp contrasts (edges) between the white and the black bars. These edges come from sinewaves with higher frequencies and lower amplitudes.
Presented below in Figure 3 are the results of a Fourier Analysis
of a square-wave grating. The results of this analysis give the frequencies and amplitudes
of the sinewaves necessary to make up the square-wave grating. This way of presenting the
results of a Fourier Analysis is called the frequency domain. It turns out that
sinewaves with frequencies that are odd multiples of the fundamental are all included in
the square-wave grating. This means that if the fundamental has a cycle width of 1",
the next sinewave will have a cycle width of 1/3", and the third will have a width of
1/5", etc. (The sequence is actually infinite). All frequencies higher than the
fundamental are referred to as harmonics. The amplitude of each sinewave is one
over the frequency. Thus, the sinewave with a frequency 11 times the fundamental will have
an amplitude of 1/11th the fundamental.
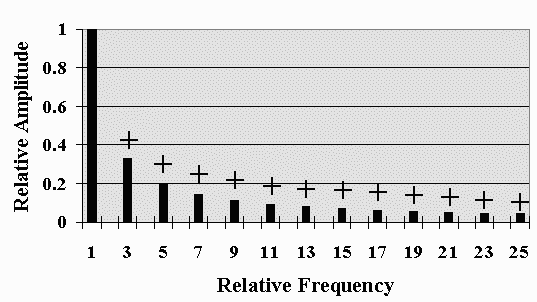
Figure 3. The results of a Fourier Analysis of a square-wave grating.
Clicking on each bar in Figure 3 will present what each individual component sinewave looks like along with the fundamental. Clicking on the plus sign just above each bar will show with the grating that is the addition of that frequency to all lower frequencies looks like. Carefully examine these frequencies before going on to answer some related questions.

 Click here to return to the beginning of
this tutorial.
Click here to return to the beginning of
this tutorial.